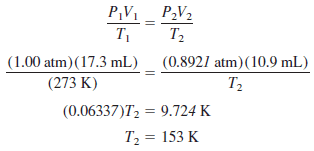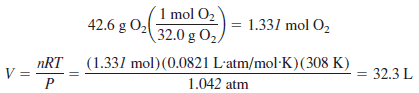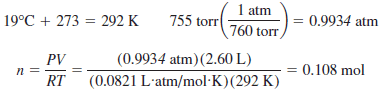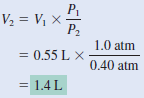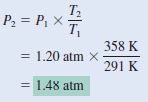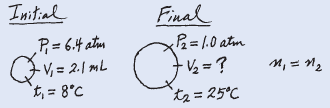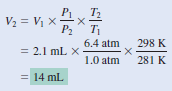The Ideal gas law: Solved Problems

– In this subject, we will discuss Solved Problems on The Ideal gas law 

Problem (1) on The ideal gas law

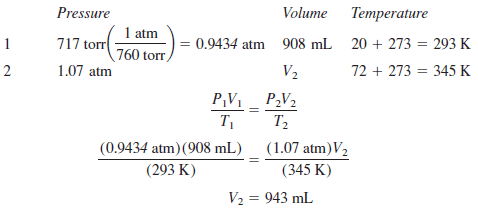
 Calculate the volume of a sample of gas originally occupying 908 mL at 717 torr and 20 ^oC after its temperature and pressure are changed to 72 ^oC and 1.07 atm.

Solution

– Again, tabulating the data is a good idea.

– The volume can be stated in milliliters in both states.

– The pressure can be stated in atmospheres in both.

– The temperature must be in kelvins in both states; it cannot merely be in the same unit in both states.

Problem (2) on The ideal gas law

A 17.3-mL sample of gas originally at standard temperature and pressure is changed to 10.9 mL at 678 torr. Calculate its final temperature in degrees Celsius.

Solution:

– The problem requires the answer in Celsius:

Problem (3) on The ideal gas law

Calculate the volume of 1.63 mol of carbon dioxide gas at 295 K and 1.14 atm.

Solution

Problem (4) on The ideal gas law

Calculate the volume of 42.6 g of oxygen gas at 35 ^oC and 792 torr.

Solution

– Because the value of R given previously has units of liters, atmospheres, moles, and kelvins, the data given here are converted to these units:

Problem (5)

 Decide which gas law should be used to solve each of the following:

(a) Calculate the final volume of a sample of gas that has an initial volume of 7.10 L at STP if the temperature and pressure are changed to 33 ^oC and 696 torr.

(b) Calculate the volume of 0.977 mol of gas at 33 ^oC and 792 torr.

Solution

(a) The combined gas law can be used.

(b) This problem involves moles and must be solved with the ideal gas law

Problem (6)

Calculate the volume of 12.7 g of water at 25 ^oC and 1.00 atm.

Solution

– Under these conditions, water is not a gas, and the ideal gas law cannot be used.

– The density of liquid water is 1.00 g/mL, and thus the volume is 12.7 mL.

– Not only the laws but also when to use each one, must be learned.

Problem (7)

Calculate the pressure of 0.0789 mol of chlorine gas that occupies 891 mL at -15°C.

Solution

– The quantities given are converted to the units generally used with the ideal gas law equation.

– Note that the nature of the gas is immaterial as long as the number of moles is known.

– The pressure is then calculated from the ideal gas law equation:

Problem (8) on The ideal gas law

Calculate the number of moles of oxygen gas in a 2.60-L container at 19 ^oC and 755 torr.

Problem (9) on The ideal gas law

 An inflated helium balloon with a volume of 0.55 L at sea level (1.0 atm) is allowed to rise to a height of 6.5 km, where the pressure is about 0.40 atm. Assuming that the temperature remains constant, what is the final volume of the balloon?

solution:

– We start with Equation:

– Because n₁ = n₂ and T₁ = T₂,

– which is Boyle’s law.

– The given information is tabulated:

Solution:

– from combined gas law:

– Because n₁ = n₂ and V₁ = V₂ , the Equation becomes:

– which is Charles’s law.  Next, we write

Problem (11)

 A small bubble rises from the bottom of a lake, where the temperature and pressure are 8^oC and 6.4 atm, to the water’s surface, where the temperature is 25^oC and the pressure is 1.0 atm. Calculate the final volume (in mL) of the bubble if its initial volume was 2.1 mL.

Solution:

 According to the combined gas law Equation:

– We assume that the amount of air in the bubble remains constant, that is, n₁ = n₂ so that

– The given information is summarized:

• Chemistry / Raymond Chang, Williams College /(10th edition).
• Fundamentals of Chemistry / David E.Goldberg/(5th edition).