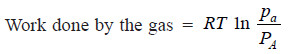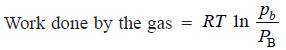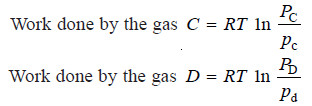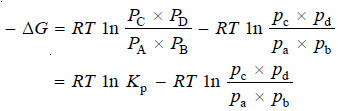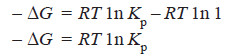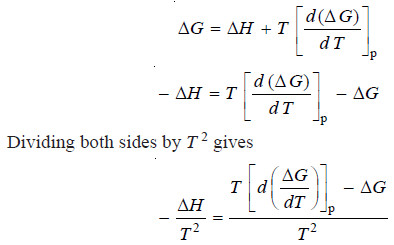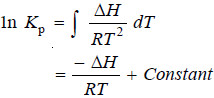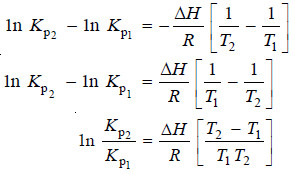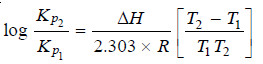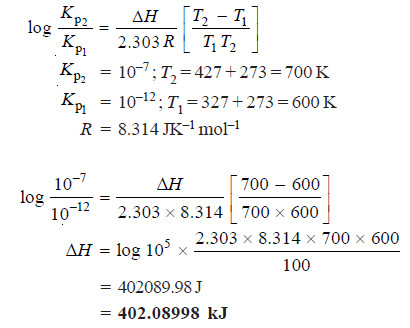Van’t Hoff isotherm – Van’t Hoff Isochore

Van’t Hoff isotherm

– The van’t Hoff isotherm gives the net work that can be obtained from a gaseous reactant at constant temperature when both the reactants and the products are at suitable arbitrary pressures.

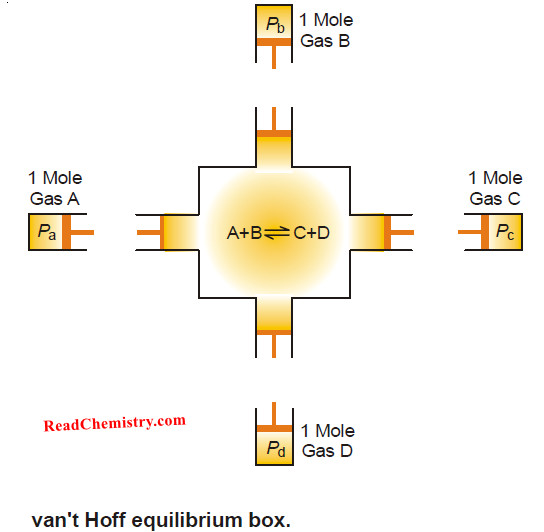
– It can be derived by using the “equilibrium box” which is a theoretical device with the supposition that of its four walls, one is permeable to A, the second to B, the third to C and the fourth to D when the gaseous reaction to be considered is

A + B ↔ C + D

– Let the initial pressures of A and B be p_a and p_b and the final pressure of C and D be p_c and p_d respectively and let the equilibrium pressure of the four be P_A, P_B, P_C and P_D respectively.

– The following theoretical operations may be performed:

(1) Change the pressure on A from the initial pressure p_a to the equilibrium pressure P_A.

(2) Change the pressure on B from pb to P_B.

(3) Introduce 1 gm mole of A and 1 gm mole of the gas B through their respective semipermeable membranes into the equilibrium box which contains the reactants and the products at the equilibrium pressure.

– This will not involve any work as the partial pressures of A and B inside the box are equal to the pressure of the gases coming in.

– A and B react to form 1 gm mole of C and 1 gm mole of D.

(4) Withdraw 1 gm mole of C and 1 gm mole of D from the equilibrium box through their respective semipermeable walls.

– No work is done in this process as the gases come out at the equilibrium pressure P_C and P_D.

(5) Now alter the pressure on the gas from the equilibrium pressure P_C and P_D to the final pressure p_c and p_d.

– As the change in free energy is equal to the total work done by the gases:

– If the reaction is started with reactants at a partial pressure of 1 atmosphere and the resulting products are also at 1 atmosphere pressure we have,

i.e., the net work of the reaction is equal to the decrease in free energy of the system and is given by the expression, RT ln K_p or 2.303 RT log K_p.

– It will be observed that ΔG is + ve when K_p is less than unity.

– It is – ve when K_p is greater than one and is zero when K_p = 1.

Van’t Hoff Isochore

– The van’t Hoff Isochore is obtained by combining the van’t Hoff Isotherm with the Gibbs Helmholtz equation.

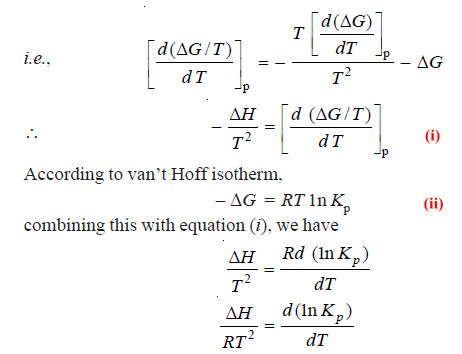
– The right-hand side of the above expression is obtained by differentiating ΔG/T w.r.t. T at constant pressure:

– The above equation is known as van’t Hoff Isochore.

– For applying the Isochore to any particular reaction, it is essential to integrate it.

– If ΔH remains constant over a range of temperature, we have on integration:

– Applying the limits T₁ and T₂ at which the equilibrium constants are K_p1 and K_p2 respectively, we have:

or

– Knowing the equilibrium constant at two different temperatures it is possible, therefore, to calculate the change in heat content.

Solved problem

The equilibrium constant Kp for a reaction A + B ↔ C + D is 10^–12 at 327^ºC and 10^–7 at 427ºC. Calculate the enthalpy of the reaction. (R = 8.314 JK^– mol^–)

Solution

Applying van’t Hoff’s equation

References