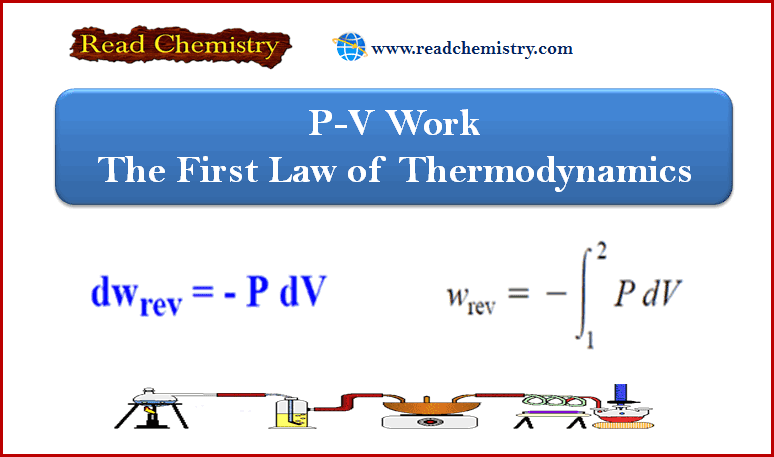
P-V work – The First Law of Thermodynamics
– In this subject, we will discuss Pressure-volume work – P-V work – the First Law of Thermodynamics
Work
– Work in thermodynamics is defined as in classical mechanics.
– When part of the surroundings exerts a macroscopically measurable force F on matter in the system while this matter moves a distance dx at the point of application of F, then the surroundings have done work on the system:
dw = Fx dx (1)
– where Fx is the component of F in the direction of the displacement.
– F may be a mechanical, electrical, or magnetic force and may act on and displace the entire system or only a part of the system.
– When Fx and the displacement dx are in the same direction, positive work is done on the system: dw > 0.
– When Fx and dx are in opposite directions, dw is negative: dw < 0
Reversible P-V Work
(a) The most common way work is done on a thermodynamic system is by a change in the system’s volume.
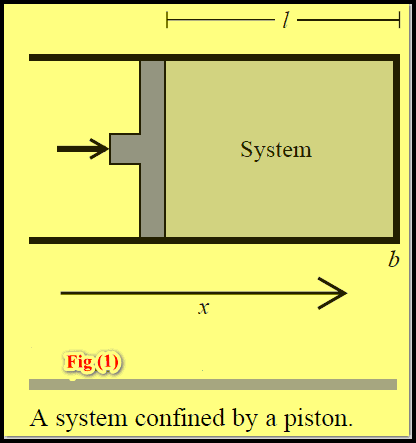
– Consider the system of Fig(1):
– The system consists of the matter contained within the piston and cylinder walls and has pressure P
– Let the external pressure on the frictionless piston also be P.
– Equal opposing forces act on the piston, and it is in mechanical equilibrium.
– Let x denote the piston’s location.
– If the external pressure on the piston is now increased by an infinitesimal amount, this increase will produce an infinitesimal imbalance in forces on the piston.
– The piston will move inward by an infinitesimal distance dx, thereby decreasing the system’s volume and increasing its pressure until the system pressure again balances the external pressure.
– During this infinitesimal process, which occurs at an infinitesimal rate, the system will be infinitesimally close to equilibrium.
– The piston, which is part of the surroundings, exerted a force, which we denote by Fx, on matter in the system at the system–piston boundary while this matter moved a distance dx.
– The surroundings therefore did work dw = Fx dx on the system.
– Let F be the magnitude of the force exerted by the system on the piston.
– Newton’s third law (action = reaction) gives F = Fx.
– The definition P = F/A of the system’s pressure P gives Fx = F = PA, where A is the piston’s cross-sectional area.
– Therefore the work dw = Fx dx done on the system in Fig (1) is:
dw = PA dx (2)
– The system has cross-sectional area A and length l = b – x (Fig 1), where x is the piston’s position and b is the position of the fixed end of the system.
– The volume of this cylindrical system is V = Al = Ab – Ax.
– The change in system volume when the piston moves by dx is dV = d(Ab – Ax) = – A dx.
– Equation (2) becomes:
dwrev = – P dV (3)
closed system, reversible process
– The subscript(rev) stands for reversible.
(b) The meaning of “reversible” will be discussed shortly.
– We implicitly assumed a closed system in deriving (equation 3).
– When the matter is transported between the system and surroundings, the meaning of work becomes ambiguous; we shall not consider this case.
– We derived (equation 3) for a particular shape of the system, but it can be shown to be valid for every system shape.
(c) We derived (3) by considering a contraction of the system’s volume (dV < 0).
– For an expansion (dV > 0), the piston moves outward (in the negative x direction), and the displacement dx of the matter at the system–piston boundary is negative (dx < 0).
– Since Fx is positive (the force exerted by the piston on the system is in the positive x direction), the work dw = Fx dx done on the system by the surroundings is negative when the system expands.
– For an expansion, the system’s volume change is still given by dV = – A dx (where dx < 0 and dV > 0), and ( equation 3) still holds.
(d) In a contraction, the work done on the system is positive (dw > 0). In an expansion, the work done on the system is negative (dw < 0). (In an expansion, the work done on the surroundings is positive.)
(e) So far we have considered only an infinitesimal volume change.
– Suppose we carry out an infinite number of successive infinitesimal changes in the external pressure.
– At each such change, the system’s volume changes by dV and work – P dV is done on the system, where P is the current value of the system’s pressure.
– The total work w done on the system is the sum of the infinitesimal amounts of work, and this sum of infinitesimal quantities is the following definite integral:
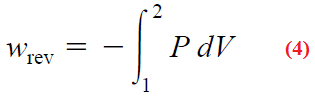
– where 1 and 2 are the initial and final states of the system, respectively.
(f) The finite volume change to which (equation 4) applies consists of an infinite number of infinitesimal steps and takes an infinite amount of time to carry out.
– In this process, the difference between the pressures on the two sides of the piston is always infinitesimally small, so finite unbalanced forces never act and the system remains infinitesimally close to equilibrium throughout the process.
– Moreover, the process can be reversed at any stage by an infinitesimal change in conditions, namely, by infinitesimally changing the external pressure.
– Reversal of the process will restore both the system and surroundings to their initial conditions.
Summary of reversible P-V Work
– A reversible process is one where the system is always infinitesimally close to equilibrium, and an infinitesimal change in conditions can reverse the process to restore both the system and surroundings to their initial states. A reversible process is an idealization.
– Equations (3) and (4) apply only to reversible expansions and contractions.
– More precisely, they apply to mechanically reversible volume changes.
– There could be a chemically irreversible process, such as a chemical reaction, occurring in the system during the expansion, but so long as the mechanical forces are only infinitesimally unbalanced, (3) and (4) apply.
– The work (equation 4) done in a volume change is called P-V work.
– Later on, we shall deal with electrical work and work changing the system’s surface area, but for now, only systems with P-V work will be considered.
– We have defined the symbol w to stand for work done on the system by the surroundings.
– Some texts use w to mean work done by the system on its surroundings. Their w is the negative of ours.
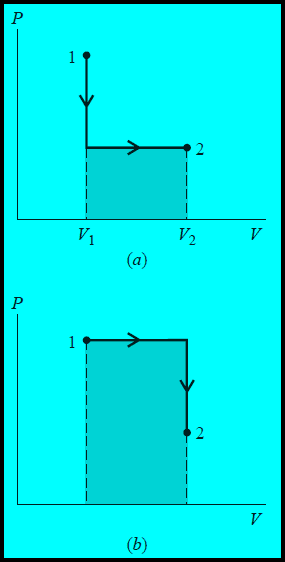
Example: Find the work wrev for processes (a) and (b) of Fig. blow if P1 = 3.00 atm, V1 = 500 cm3, P2 = 1.00 atm, and V2 = 2000 cm3. Also, find wrev for the reverse of the process (a).
Solution:
Irreversible P-V Work
– The work w in a mechanically irreversible volume change sometimes cannot be calculated with thermodynamics.
– For example, suppose the external pressure on the piston in Fig.(1) is suddenly reduced by a finite amount and is held fixed thereafter.
– The inner pressure on the piston is then greater than the outer pressure by a finite amount, and the piston is accelerated outward.
– This initial acceleration of the piston away from the system will destroy the uniform pressure in the enclosed gas.
– The system’s pressure will be lower near the piston than farther away from it.
– Moreover, the piston’s acceleration produces turbulence in the gas.
– Thus we cannot give a thermodynamic description of the state of the system.
– We have dw = Fx dx. For P-V work, Fx is the force at the system–surroundings boundary, which is where the displacement dx is occurring.
– This boundary is the inner face of the piston, so dwirrev = – Psurf dV, where Psurf is the pressure the system exerts on the inner face of the piston. (By Newton’s third law, Psurf is also the pressure the piston’s inner face exerts on the system.)
– Because we cannot use thermodynamics to calculate Psurf during the turbulent, irreversible expansion, we cannot find dwirrev from thermodynamics.
– The law of conservation of energy can be used to show that, for a frictionless piston,
dwirrev = – Pext dV – dKpist (5)
– Pext is the external pressure on the outer face of the piston
– dKpist is the infinitesimal change in piston kinetic energy.
– The integrated form of (equation 5) is :
– If we wait long enough, the piston’s kinetic energy will be dissipated by the internal friction (viscosity) in the gas.
– The gas will be heated, and the piston will eventually come to rest (perhaps after undergoing oscillations).
– Once the piston has come to rest, we have ΔKpist = 0 – 0 = 0, since the piston started and ended at rest.
– We then have:
– Hence we can find wirrev after the piston has come to rest.
– If, however, part of the piston’s kinetic energy is transferred to some other body in the surroundings before the piston comes to rest, then thermodynamics cannot calculate the work exchanged between the system and surroundings.
Summary of Pressure-Volume Work
– For now, we shall deal only with work done due to a volume change.
– The work done on a closed system in an infinitesimal mechanically reversible process is:
dwrev = -P dV
– The work:

depends on the path (the process) used to go from the initial state 1 to the final state 2.
Reference: Physical Chemistry /Ira N. Levine / University of New York /6th .ed./ 2002.