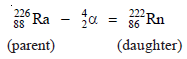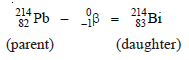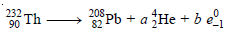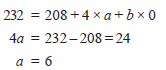Radioactive decay: Definition, Types, Examples

– In this subject, we will discuss the Radioactive decay: Definition, Types, Examples

– According to the theory put forward by Rutherford and Soddy (1903), radioactivity is a nuclear property.

– The nucleus of a radioactive atom is unstable.

– It undergoes decay or disintegration by spontaneous emission of an α- or β-particle.

– This results in the change of proton-neutron composition of the nucleus to form a more stable nucleus.

– The original nucleus is called the parent nucleus and the product is called the daughter nucleus.

– As evident from above, there are two chief types of Radioactive decay: α-decay and β-decay

α-Decay

– When a radioactive nucleus decays by the emission of an α-particle (α-emission) from the nucleus, the process is termed α-decay.

– An alpha particle has four units of atomic mass and two units of positive charge.

– If Z is the atomic number and M is the atomic mass of the parent nucleus, the daughter nucleus will have:

– Thus an α-emission reduces the atomic mass by 4 and atomic number by 2.

– For example, Radium decays by α-emission to form a new element Radon,

β-Decay

– When a radioactive nucleus decays by β-particle emission (β-emission), it is called β-decay.

– A free β-particle or electron does not exist as such in the nucleus.

– It is produced by the conversion of a neutron to a proton at the moment of emission.

– This results in the increase of one positive charge on the nucleus.

– The loss of a β-particle from the nucleus does not alter its atomic mass.

– For a parent nucleus with atomic mass M and atomic number Z, the daughter nucleus will have:

– Thus a β-emission increases the atomic number by 1 with no change in atomic mass.

– An example of β-decay is the conversion of lead-214 to bismuth-214,

– It is noteworthy that a β-emission results in the production of an isobar.

– Thus, ²¹⁴Pb₈₂ and ²¹⁴Bi₈₃ are isobaric as they have the same mass number 214 but different atomic numbers (82 and 83).

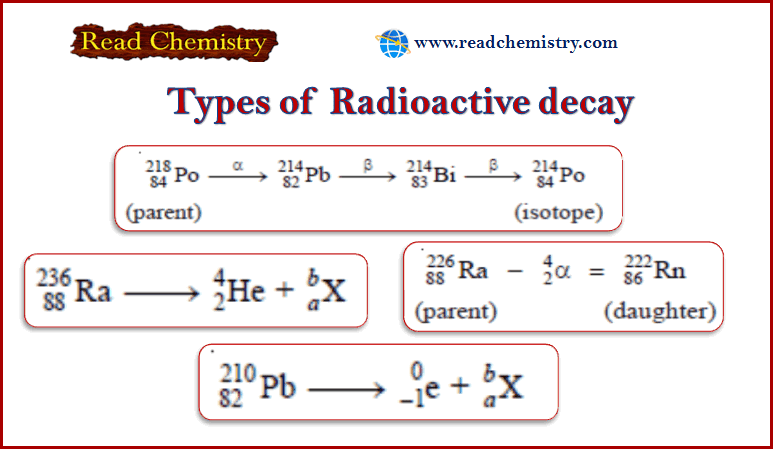
– One α-emission and two β-emissions yield an isotope.

– Let us consider the following series of changes.

– The parent element ²¹⁸Po₈₄ emits an α-particle and subsequently, two β-particles, resulting in the formation of ²¹⁴Po₈₄which is an isotope of the parent.

– Both the parent and the end-product have the same atomic number 84 but different mass numbers (218 and 214).

Solved Problems on Radioactive decay

Problem (1): How many α and β particles are emitted in passing down from ²³²Th₉₀ to ²⁰⁸Pb₈₂?

Solution:

– Let (a) be the number of α particles and (b) be the number of β particles emitted during the radioactive transformation.

– It can be represented as:

– Comparing the mass numbers, we get

– Comparing the atomic numbers, we get

– Substituting the value of a, we get

– Thus the number of α particles emitted = 6 and the number of β particles emitted = 4

Problem (2): ²¹⁰Pb₈₂ is a β-emitter and ²²⁶Ra₈₈ is anα-emitter. What will be the atomic masses and atomic numbers of daughter elements of these radioactive elements? Predict the position of daughter elements in the periodic table.

Solution:

(a) ²¹⁰Pb₈₂ undergoes β-decay i.e.

– Comparing the atomic masses, we have

– and comparing the atomic numbers, we get

– Thus the daughter element will have the same atomic mass 210 and its atomic number will be 83.

– It will occupy one position right to the parent element.

(b) ²³⁶Ra₈₈ undergoes α decay i.e.

– Comparing the atomic masses, we get:

– and comparing the atomic number, we get:

– Thus the daughter element will have an atomic mass of 232 and its atomic number will be 86.

– It will occupy two positions to the left of the parent element.

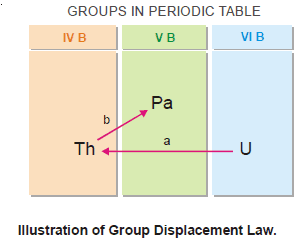
The group displacement law

– The position number of an element in a Group of the Periodic Table corresponds to its atomic number.

– If the atomic number of a given element is changed, its Group also changes accordingly.

– We know that an α-emission decreases the atomic number of the parent element by 2 and a β emission increases the atomic number by 1.

– Thus: in an α-emission, the parent element will be displaced to a Group two places to the left and in a β-emission, it will be displaced to a Group One place to the right.

– This is called the Group Displacement Law.

– It was first stated by Fajans and Soddy (1913) and is often named after them as (Fajans-Soddy Group Displacement Law).

Reference: Essentials of Physical Chemistry /Arun Bahl, B.S Bahl and G.D. Tuli / multicolor edition.