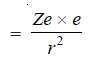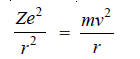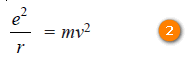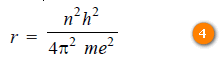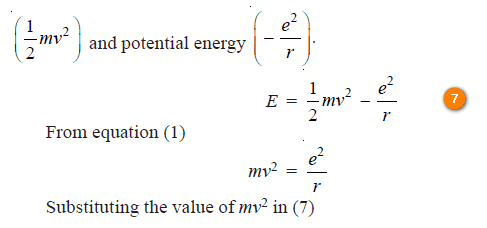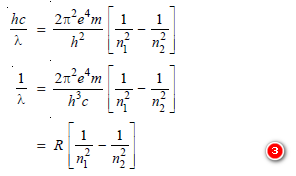Bohr Model of atom – Bohr Theory

– Bohr theory was based on Planck’s quantum theory and was built on some postulates.

– Rutherford’s nuclear model simply stated that atom had a nucleus and the negative electrons were present outside the nucleus.

– It did not say anything as to how and where those electrons were arranged.

– It also could not explain why electrons did not fall into the nucleus due to electrostatic attraction.

– In 1913 Niels Bohr proposed a new model of atom which explained some of these things and also the emission spectrum of hydrogen.

– Bohr theory was based on Planck’s quantum theory and was built on the following postulates as follow:

Postulates of Bohr Theory

Postulates (1):

Electrons travel around the nucleus in specific permitted circular orbits and in no others.

– Electrons in each orbit have a definite energy and are at a fixed distance from the nucleus.

– The orbits are given the letter designation (n) and each is numbered 1, 2, 3, etc. (or K, L, M, etc.) as the distance from the nucleus increases.

Postulates (2)

While in these specific orbits, an electron does not radiate (or lose) energy.

– Therefore in each of these orbits the energy of an electron remains the same i.e. it neither loses nor gains energy.

– Hence the specific orbits available to the electron in an atom are referred to as stationary energy levels or simply energy levels.

Postulates (3)

An electron can move from one energy level to another by quantum or photon jumps only.

– When an electron resides in the orbit which is lowest in energy (which is also closest to the nucleus), the electron is said to be in the ground state.

– When an electron is supplied energy, it absorbs one quantum or photon of energy and jumps to a higher energy level.

– The electron then has potential energy and is said to be in an excited state.

– The quantum or photon of energy absorbed or emitted is the difference between the lower and higher energy levels of the atom.

– where (h) is Planck’s constant and (ν) is the frequency of a photon emitted or absorbed energy.

Postulates (4)

The angular momentum (mvr) of an electron orbiting around the nucleus is an integral multiple of Planck’s constant divided by 2π.

• m = mass of electron,
• v = velocity of the electron,
• r = radius of the orbit ;
• n = 1, 2, 3, etc.,
• h = Planck’s constant.

– By putting the values 1, 2, 3, etc., for (n), we can have respectively the angular momentum

– There can be no fractional value of h/2π.

– Thus the angular momentum is said to be quantized.

– The integer n in equation (2) can be used to designate an orbit and a corresponding energy level n is called the atom’s Principal quantum number.

– Using the above postulates and some classical laws of Physics, Bohr was able to calculate the radius of each orbit of the hydrogen atom, the energy associated with each orbit, and the wavelength of the radiation emitted in transitions between orbits.

– The wavelengths calculated by this method were found to be in excellent agreement with those in the actual spectrum of hydrogen, which was a big success for the Bohr model.

Calculation of radius of orbits

– Consider an electron of charge e revolving around a nucleus of charge (Ze), 

where:

• (Z) is the atomic number
• (e) the charge on a proton.
• (m) be the mass of the electron,
• (r) the radius of the orbit
• (ν) the tangential velocity of the revolving electron.

– The electrostatic force of attraction between the nucleus and the electron (Coulomb’s law),

– The centrifugal force acting on the electron:

– Bohr assumed that these two opposing forces must be balancing each other exactly to keep the electron in orbit.

Thus

– For hydrogen Z = 1, therefore,

– Multiplying both sides by (r)

– According to one of the postulates of Bohr’s theory, the angular momentum of the revolving electron is given by the expression:

– Substituting the value of ν in equation (2),

– Solving for (r),

– Since the value of h, m and e had been determined experimentally, substituting these values in (4), we have:

where n is the principal quantum number and hence the number of the orbit.

– When n = 1, the equation (5) becomes:

– This last quantity, α₀ called the first Bohr radius was taken by Bohr to be the radius of the hydrogen atom in the ground state.

– This value is reasonably consistent with other information on the size of atoms.

– When n = 2, 3, 4, etc., the value of the second and third orbits of hydrogen comprising the electron in the excited state can be calculated.

Solved Problem 

Calculate the first five Bohr radii.

Solution

– The equation (5) may be written as:

The energy of electron in each orbit

– For the hydrogen atom, the energy of the revolving electron, (E) is the sum of its kinetic energy

Solved Problem

Calculate the five lowest energy levels of the hydrogen atom.

Solution

– From equation (10)

– Therefore the energy associated with the first five energy levels (or orbits) is:

Significance of Negative Value of Energy

– The energy of an electron at infinity is arbitrarily assumed to be zero.

– This state is called zero-energy state.

– When an electron moves and comes under the influence of nucleus, it does some work and spends its energy in this process.

– Thus the energy of the electron decreases and it becomes less than zero i.e. it acquires a negative value.

Bohr Explanation of Hydrogen Spectrum

– The solitary electron in hydrogen atom at ordinary temperature resides in the first orbit (n = 1) and is in the lowest energy state (ground state).

– When energy is supplied to hydrogen gas in the discharge tube, the electron moves to higher energy levels viz., 2, 3, 4, 5, 6, 7, etc., depending on the quantity of energy absorbed.

– From these high energy levels, the electron returns by jumps to one or other lower energy level.

– In doing so the electron emits the excess energy as a photon.

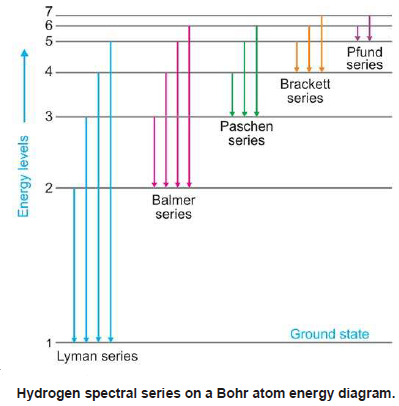
– This gives an excellent explanation of the various spectral series of hydrogen.

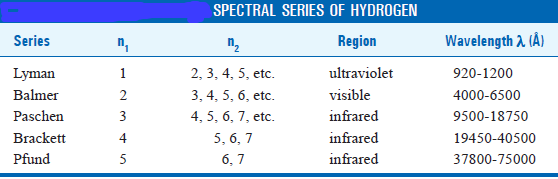
– Lyman series is obtained when the electron returns to the ground state i.e., n = 1 from higher energy levels (n₂ = 2, 3, 4, 5, etc.).

– Similarly, Balmer, Paschen, Brackett, and Pfund series are produced when the electron returns to the second, third, fourth, and fifth energy levels respectively as shown in the following figure:

– The value of Rydberg’s constant is the same as in the original empirical Balmer’s equation.

– According to equation (1), the energy of the electron in orbit n₁ (lower) and n₂ (higher) is

– The difference of energy between the levels n₁ and n₂ is :

– According to Planck’s equation:

– where λ is the wavelength of the photon and (c) is the velocity of light.

– From equation (1) and (2), we can write:

– where R is the Rydberg constant.

– The value of R can be calculated as the value of (e, m, h, and c) are known.

– It comes out to be 109,679 cm^–1 and agrees closely with the value of the Rydberg constant in the original empirical Balmer’s equation (109,677 cm^–1). 

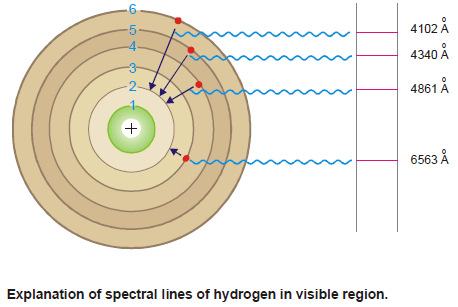
Calculation of wavelengths of the spectral lines of Hydrogen in the visible region

– These lines constitute the Balmer series when n₁ = 2. Now the equation (3) above can be written as:

– Thus the wavelengths of the photons emitted as the electron returns from energy levels 6, 5, 4, and 3 were calculated by Bohr.

– The calculated values corresponded exactly to the values of wavelengths of the spectral lines already known.

– This was, in fact, a great success of the Bohr atom.

Solved Problem

Find the wavelength in Å of the line in Balmer series that is associated with drop of the electron from the fourth orbit. The value of Rydberg constant is 109,676 cm^–1.

Solution

– The wavelengths of lines in Balmer series are given by:

– where λ = wavelength, R (Rydberg constant) = 109,676 cm^–1 ; n = 4.

– The wavelength of the spectral line is 6561 Å

Shortcomings of The Bohr atom

(1) The great success of the Bohr theory was in its ability to predict lines in the hydrogen atom spectrum.

– But it was spectacularly unsuccessful for every other atom containing more than one electron.

(2) We no longer believe in well-defined electron orbits as was assumed by Bohr.

– In fact, in view of modern advances, like dual nature of matter, the uncertainty principle, any mechanical model of the atom stands rejected.

(3) Bohr’s model of electronic structure could not account for the ability of atoms to form molecules through chemical bonds.

– Today we only accept Bohr’s views regarding quantization as nobody has explained atomic spectra without numerical quantization and no longer attempted description of atoms on classical mechanics.

(4) Bohr’s theory could not explain the effect of magnetic field (Zeeman effect) and electric field (Stark effect) on the spectra of atoms.

Sommerfeld’s Modification of Bohr atom

– When spectra were examined with spectrometers, each line was found to consist of several closely packed lines.

– The existence of these multiple spectral lines could not be explained on the basis of Bohr’s theory.

– Sommerfeld modified Bohr’s theory as follows.

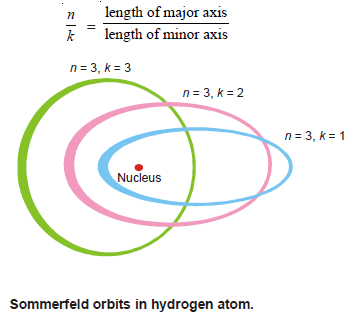
– Bohr considered electron orbits as circular but Sommerfeld postulated the presence of elliptic orbits also.

– An ellipse has a major and minor axis.

– A circle is a special case of an ellipse with equal major and minor axis.

– The angular momentum of an electron moving in an elliptic orbit is also supposed to be quantized.

– Thus only a definite set of values is permissible.

– It is further assumed that the angular momentum can be an integral part of (h/2π) units, where h is Planck’s constant. Or that,

– where (k) is called the azimuthal quantum number, whereas the quantum number used in Bohr’s theory is called the principal quantum number.

– The two quantum numbers n and k are related by the expression:

– The values of (k) for a given value of (n) are k = n – 1, n – 2, n – 3, and so on.

– A series of elliptic orbits with different eccentricities result for the different values of k.

– When n = k, the orbit will be circular. In other words (k) will have (n) possible values (n to 1) for a given value of (n).

– However, calculations based on wave mechanics have shown that this is incorrect and Sommerfeld’s modification of the Bohr atom fell through.

Reference: Essentials of Physical Chemistry /Arun Bahl, B.S Bahl and G.D. Tuli / multicolour edition.