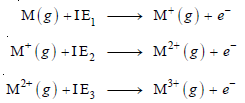Ionization Energy (Definition – Trends – Measurement)

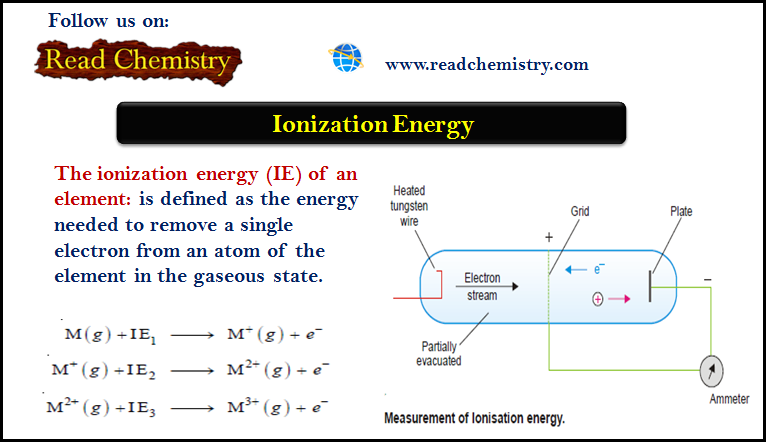
– The ionization energy (IE) of an element is defined as the energy needed to remove a single electron from an atom of the element in the gaseous state.

Ionization Energy

– The process of removing an electron from an isolated atom to form a positive ion is called ionization.

– Energy will be required to remove an electron from the atom against the force of attraction of the nucleus.

– The ionization energy (IE) of an element is defined as the energy needed to remove a single electron from an atom of the element in the gaseous state.

That is,

– Since one, two, or more electrons may be removed from the same atom, one after the other, we have as many ionization energies of the element.

– The First ionization energy (IE₁), is the energy needed to remove the first electron from the gaseous atom M to form M+ ion.

– The Second ionization energy (IE₂), is the energy needed to remove a second electron, from the gaseous M⁺ ion to form M²⁺ ion.

– Higher ionization energies can be defined in the same way.

– We can depict the first, second, and third ionization energies in the form of equations:

– Ionization energies are sometimes called Ionization potentials.

– Ionization energies are usually expressed in electron volts (eV) per atom, or in kilojoules per mole of atoms (kJ mol^–1).

– For conversion, 1eV atom^–1 = 96.48 kJ mol^–1.

Measurement of Ionisation Energy

– The amount of energy required to detach an electron from an atom can be measured by supplying the required energy as thermal energy, electrical energy, or radiant energy.

– Thus ionization energies can be determined from the spectrum of the element or by any of the two methods detailed below.

(1) The Electrical method

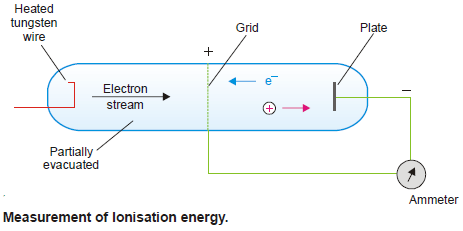
– The apparatus used is shown in the following figure:

– The electrically heated tungsten wire emits electrons.

– The grid can be charged positively to different voltages which we read with a voltmeter.

– and The plate opposite the grid has a small negative charge.

– When the potential to the grid is zero, no current flows between the grid and the plate.

– However, if we give sufficient potential to the grid, the electrons emitted by the tungsten wire are accelerated towards the grid, pass through it and ionize the atoms between the grid and plate.

– The electron ejected by each atom is attracted to grid and positive ion is attracted to plate.

– A current thus passes between grid and plate which is shown up by an ammeter.

– The minimum grid voltage that just produces a current is called ionization potential.

– If V be the ionization potential, the ionization energy (IE) is calculated as:

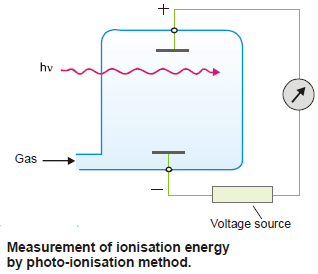
(2) Photo-ionisation Method

– The gaseous atoms are introduced into a chamber containing two electrically charged plates

– As neutral atoms, they do not conduct electricity, and no current flows between the plates.

– When radiant energy (hν) is supplied to the gaseous atoms, ionization will occur and electric current will flow.

– The frequency of the radiation used is gradually increased.

– The minimum frequency necessary to cause ionization of the gaseous atoms, as shown by the flow of an electric current is noted.

– From this frequency, the ionization energy is calculated.

Order of Successive Ionization Energy

– The second ionization energy (IE₂) is larger than the first ionization energy (IE₁) because it is more difficult to detach an electron from a +ve ion than a neutral atom.

– The third ionization energy (IE₃) is still larger as the third electron has to be detached from a 2+ ion.

– Thus in general successive ionisation energies increase in magnitude. That is,

IE₁< IE₂ < IE₃ < IE₄, and so on.

– For illustration, the first four ionization energies for sodium and magnesium are listed below:

Principal Trends in Ionization Energy

– A graph of the first ionization energies against atomic number (Z) for the first 18 elements of the Periodic Table is shown in the following figure:

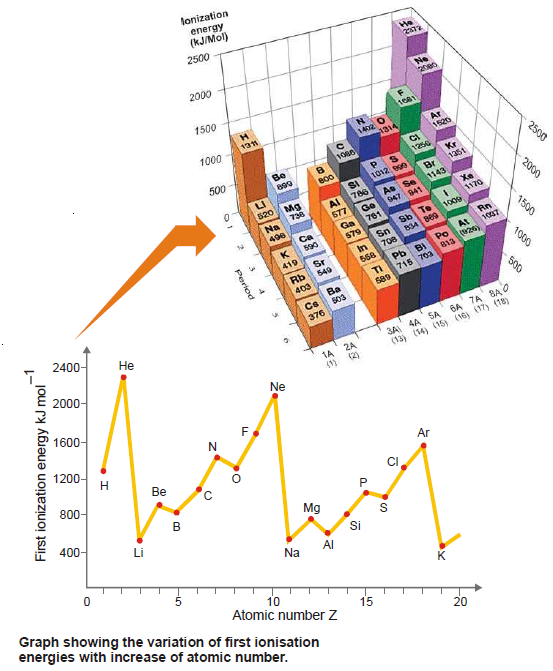
– The important trends as illustrated by the graph are:

(1) Ionisation energies increase across a period. e.g., Li to Ne.

(2) Ionisation energies decrease down a group e.g., Li, Na, K.

(3) There are regular discontinuities in the increase trend across a period e.g., Be to B, and N to O.

(1) Increase across a Period

– As we pass from left to right in a period, the first ionization energy shows a steady increase.

– Thus in Period 2 from Li to N, we have:

Explanation

– The outer-shell electrons in the elements of the same period are arranged in the same shell.

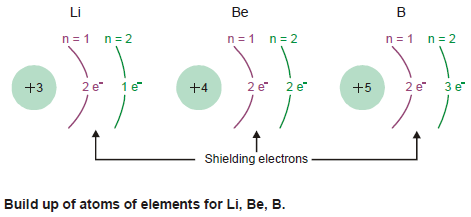
– For example, the build up of electrons in Period 2 from Li to B is shown in the following figure:

– Moving from Li to B, the positive charge on the nucleus increases whereas the distance between the nucleus and valence electrons decreases.

– Therefore more energy is required to remove an electron as we go from left to right in the Period.

– Since the number of screening electrons remains the same, they do not upset the increase trend.

(2) Decrease down a Group

– In the elements of a vertical Group of the Periodic table, the number of outer shell electrons is the same.

– But the following changes are noted from top to bottom.

(1) The principal quantum number n containing the valence electrons increases.

(2) The nuclear charge (At. No.) increases.

(3) The number of electrons in the inner shells (shielding electrons) increases.

– The net result of these changes is that the first ionization energies down a group record a progressive decrease.

– Thus for Group IA, we have:

– Let us explain the above decrease trend by taking example of lithium and sodium.

– They have the atomic structures

– Lithium and sodium both have one outer-shell electron.

– The number of shielding electrons in sodium is 10 while in lithium it is 2.

– If we assume that the inner shell electrons provide hundred percent screening, the core charge attracting the outer-shell electron would be :

– Thus the same net charge (+ 1) attracts the outer-shell electrons to the core.

– But the distance of the outer electron from the nucleus is greater in Na (n = 3) than in Li (n = 1).

– Therefore the force of attraction between the outer electron and the core will be less in Na than in Li.

– That explains the lower IE of Na compared to Li.

– By the same line of argument, the decrease trend in IE from element to element while going down a Group can be justified.

(3) Regular Discontinuities

– As already discussed, the first ionization energies increase across a period.

– But this increase trend is upset at the third and sixth element in a period.

– As clear from the graph in Fig.(1), there are breaks at B and O which occupy the third and fifth positions respectively in the 2nd period.

– The IE₁ of B is less than that of Be and the IE₁ of O is less than that of N.

Explanation

(a) The electronic configuration of Be and B are

– The 2p orbital electron of B is already higher in energy than the 2s orbital electron.

– Therefore the removal of an electron from B requires less energy and its IE1 is lower.

(b) The electronic configuration of N and O is :

– The 2p orbitals may be represented as:

Whenever two electrons occupy a particular orbital, they repel each other.

– As a result, it is easier to remove one of the paired 2p electrons from O than it is to remove an unpaired electron from N atom.

– Thus IE₁ of O is lower than that of N.

Reference: Essentials of Physical Chemistry /Arun Bahl, B.S Bahl and G.D. Tuli / multicolor edition.