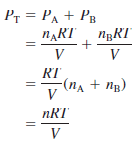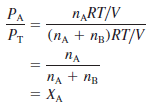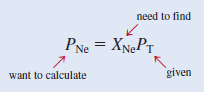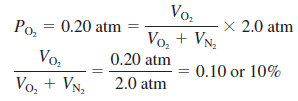Dalton’s Law of Partial Pressures (Statement, Applications)

– In this subject, we will discuss Dalton’s Law of Partial Pressures (Statement, Mathematical, Importance, Application).

Dalton’s Law of Partial Pressures

– Thus far we have concentrated on the behavior of pure gaseous substances, but experimental studies very often involve mixtures of gases.

– For example, for a study of air pollution, we may be interested in the pressure-volume temperature relationship of a sample of air, which contains several gases.

– In this case, and all cases involving mixtures of gases, the total gas pressure is related to partial pressures, that is, the pressures of individual gas components in the mixture.

– Partial pressures are the pressures of individual gas components in the mixture.

Statement of Dalton Dalton’s Law of Partial Pressures

In 1801 Dalton formulated a law, now known as Dalton’s law of partial pressures, which states that:

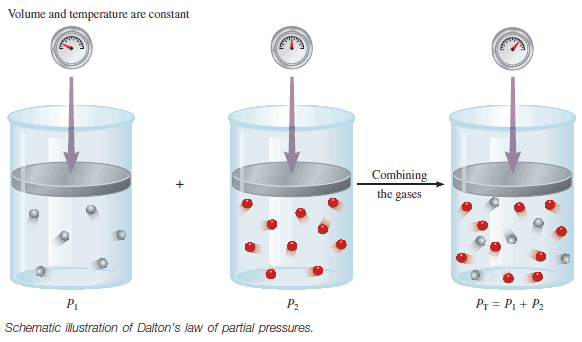
The total pressure of a mixture of gases is just the sum of the pressures that each gas would exert if it were present alone.

– The following figure illustrates Dalton’s law.

Mathematical Expression of  Dalton’s Law

– Consider a case in which two gases, A and B, are in a container of volume V.

– The pressure exerted by gas A, according to the ideal gas equation, is:

– where n_A is the number of moles of A present.

– Similarly, the pressure exerted by gas B is:

– In a mixture of gases A and B, the total pressure P_T is the result of the collisions of both types of molecules, A and B, with the walls of the container.

– Thus, according to Dalton’s law,

–  n: the total number of moles of gases present, is given by n = n_A + n_B,

– P_A and P_B: are the partial pressures of gases A and B, respectively.

– For a mixture of gases, then, P_T depends only on the total number of moles of gas present, not on the nature of the gas molecules.

– In general, the total pressure of a mixture of gases is given by:

– where P₁, P₂, P₃, . . . are the partial pressures of components 1, 2, 3, . . . .

– To see how each partial pressure is related to the total pressure, consider again the case of a mixture of two gases A and B. Dividing P_Aby P_T, we obtain:

– where X_A is called the mole fraction of A.

– The mole fraction: is a dimensionless quantity that expresses the ratio of the number of moles of one component to the number of moles of all components present.

– In general, the mole fraction of component (i) in a mixture is given by:

– where n_i and n_T are the number of moles of component i and the total number of moles present, respectively.

– The mole fraction is always smaller than 1. We can now express the partial pressure of A as:

– Similarly,

– Note that the sum of the mole fractions for a mixture of gases must be unity.

– If only two components are present, then

– If a system contains more than two gases, then the partial pressure of the i th component is related to the total pressure by:

Determination of partial pressures

– How are partial pressures determined? A manometer can measure only the total pressure of a gaseous mixture.

– To obtain the partial pressures, we need to know the mole fractions of the components, which would involve elaborate chemical analyses.

– The most direct method of measuring partial pressures is using a mass spectrometer.

– The relative intensities of the peaks in a mass spectrum are directly proportional to the amounts, and hence to the mole fractions, of the gases present.

– From mole fractions and total pressure, we can calculate the partial pressures of individual components, as solved Problem (1) shows.

Solved Problems

Problem (1): A mixture of gases contains 4.46 moles of neon (Ne), 0.74 moles of argon (Ar), and 2.15 moles of xenon (Xe). Calculate the partial pressures of the gases if the total pressure is 2.00 atm at a certain temperature.

Strategy:

What is the relationship between the partial pressure of a gas and the total gas pressure? How do we calculate the mole fraction of a gas?

Solution:

– The partial pressure of Ne (P_Ne) is equal to the product of its mole fraction (X_Ne) and the total pressure (P_T)

– we calculate the mole fraction of Ne as follows:

– Therefore:

Check:

– Make sure that the sum of the partial pressures is equal to the given total pressure; that is,

(1.21 + 0.20 + 0.586) atm = 2.00 atm. 

Importance of Dalton’s law of partial pressures

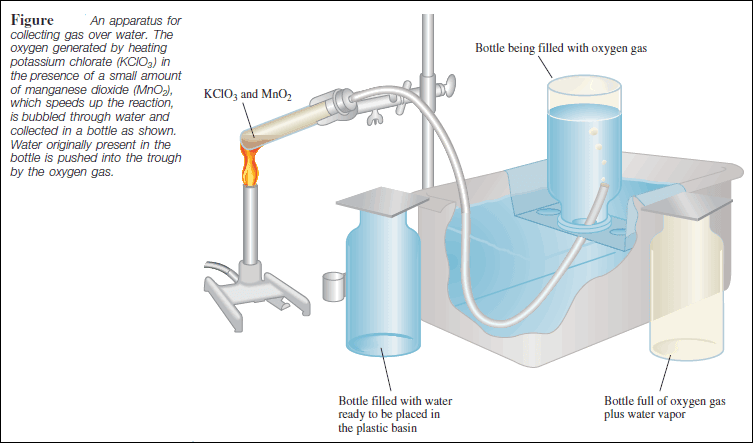
– Dalton’s law of partial pressures is useful for calculating volumes of gases collected over water.

– For example, when potassium chlorate (KClO₃ ) is heated, it decomposes to KCl and O₂ :

– The oxygen gas can be collected over water, as shown in the following Figure:

– Initially, the inverted bottle is completely filled with water.

– As oxygen gas is generated, the gas bubbles rise to the top and displace water from the bottle.

– This method of collecting a gas is based on the assumption that the gas does not react with water and that it is not appreciably soluble in it.

– These assumptions are valid for oxygen gas, but not for gases such as NH₃, which dissolves readily in water.

– The oxygen gas collected in this way is not pure, however, because water vapor is also present in the bottle.

– The total gas pressure is equal to the sum of the pressures exerted by the oxygen gas and the water vapor:

– Consequently, we must allow for the pressure caused by the presence of water vapor when we calculate the amount of O₂ generated.

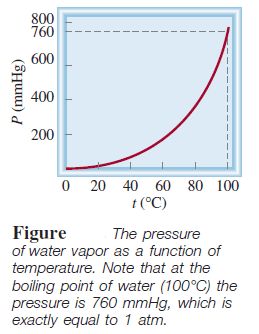
– The following Table shows the pressure of water vapor at various temperatures.

 

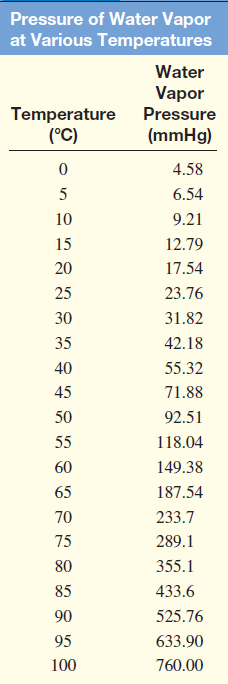
– These data are plotted in the following Figure:

– solved Problem (2) shows how to use Dalton’s law to calculate the amount of a gas collected over water.

Solved Problem

Problem (2): Oxygen gas generated by the decomposition of potassium chlorate is collected as shown in Figure (1). The volume of oxygen collected at 24 ^°C and atmospheric pressure of 762 mmHg is 128 mL. Calculate the mass (in grams) of oxygen gas obtained. The pressure of the water vapor at 24°C is 22.4 mmHg.

Strategy:

 To solve for the mass of O₂ generated, we must first calculate the partial pressure of O₂ in the mixture. What gas law do we need? How do we convert the pressure of O₂ gas to mass of O₂ in grams?

Solution:

– From Dalton’s law of partial pressures, we know that:

– From the ideal gas equation, we write:

– where m and µ are the mass of O₂ collected and the molar mass of O₂, respectively.

– Rearranging the equation we obtain

 

Check:

– The density of the oxygen gas is (0.164 g/0.128 L), or 1.28 g/L, which is a reasonable value for gases under atmospheric conditions.

Application of Dalton’s law of partial pressures (scuba diving)

– A direct application of Dalton’s law of partial pressures to scuba diving is discussed here

– Our example is a direct application of Dalton’s law.

– Oxygen gas is essential for our survival, so it is hard to believe that an excess of oxygen could be harmful. Nevertheless, the toxicity of too much oxygen is well established.

– For example, newborn infants placed in oxygen tents often sustain damage to the retinal tissue, which can cause partial or total blindness.

– Our bodies function best when oxygen gas has a partial pressure of about 0.20 atm, as it does in the air we breathe.

– The oxygen partial pressure is given by:

– where P_T is the total pressure.

– However, because volume is directly proportional to the number of moles of gas present (at constant temperature and pressure), we can now write:

– Thus, the composition of air is 20% oxygen gas and 80% nitrogen gas by volume.

– When a diver is submerged, the pressure of the water on the diver is greater than atmospheric pressure.

– The air pressure inside the body cavities (for example, lungs, sinuses) must be the same as the pressure of the surrounding water; otherwise, they would collapse.

– A special valve automatically adjusts the pressure of the air breathed from a scuba tank to ensure that the air pressure equals the water pressure at all times.

– For example, at a depth where the total pressure is 2.0 atm, the oxygen content in the air should be reduced to 10 percent by volume to maintain the same partial pressure of 0.20 atm; that is,

– Although nitrogen gas may seem to be the obvious choice to mix with oxygen gas, there is a serious problem with it.

– When the partial pressure of nitrogen gas exceeds 1 atm, enough of the gas dissolves in the blood to cause a condition known as nitrogen narcosis.

– The effects on the diver resemble those associated with alcohol intoxication.

– Divers suffering from nitrogen narcosis have been known to do strange things, such as dancing on the seafloor and chasing sharks.

– For this reason, helium is often used to dilute oxygen gas.

– An inert gas, helium is much less soluble in blood than nitrogen and produces no narcotic effects.

Reference: Chemistry / Raymond Chang, Williams College /(10th edition).