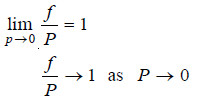Fugacity and activity

– In general, it may be stated that each substance in a given state has a tendency to escape from that state and this escaping tendency denoted by f is called fugacity.

Fugacity and Activity

– It has already been pointed out that equation ΔG = RT log_e P₂/P₁ is applicable only to ideal gases.

– When applied to real gases, particularly at higher pressures, it is found that this expression does not reproduce the change in free energy, the reason being that under these conditions V is not equal to nRT/P.

– In order to apply this equation to non-ideal systems, Lewis introduced two new thermodynamic quantities, fugacity and activity.

– Consider a system composed of liquid water and its vapour.

– Liquid water has a tendency to escape into the vapour phase while the vapour tends to escape the gaseous state and come into the liquid phase by condensation.

– When the system is in equilibrium, these two escaping tendencies become equal and we observe a constant vapour pressure at a constant temperature.

– In general, it may be stated that each substance in a given state has a tendency to escape from that state and this escaping tendency denoted by f is called fugacity.

– It is related t the free energy content (G) by the expression:

G = RT ln f + B

– where B is a constant depending upon the temperature and the nature of the substance.

– It is not possible to evaluate B since the absolute values of the free energy are not known.

– To circumvent this difficulty, all free energy measurements for any given substance are referred to as standard reference point.

– If we represent by G^º the free energy per mole and f^º the fugacity in this standard state, then Gº is given by

G^º = RT ln f^º + B

– If G is the free energy of the substance in any state, then the free energy difference between this state and the standard state is given by

– G^º = RT ln f / f^º

or    G = G^º + RT ln f / f^º …(i)

– The ratio f / f^º is called activity and is denoted by the symbol a.

– The activity of any substance may therefore, be defined as the ratio of fugacity of the substance in the given state to the fugacity of the same substance in the standard state.

G = G^º + RT ln a

– In the standard state,

G = G^º

∴ RT ln a = 0 or a = 1

i.e., in the standard state the activity of a substance is equal to unity.

– In any other state the value of activity will depend upon the difference (G – Gº).

– The difference in free energy per mole caused on passing from one state in which the free energy is G₁ and the activity a₁ to another state in which these are G₂ and a₂ respectively, is given by the expression:

ΔG = G₂ – G₁ = (G^º + RT ln a₂) – (Gº + RT ln a₁)

or

– The similarity between the above equation and equation:

ΔG = RT ln P₂/P₁

suggests that activity is the thermodynamic counterpart of the gas pressure.

– For the standard state of any gas at the given temperature, the fugacity is taken as equal to unity, viz, f ^º = 1 and on the basis of this definition the activity of any gas becomes equal to fugacity

– The equation (i) can, therefore, be written as:

G = G^º + RT log_e f

– For an ideal gas the fugacity is equal to pressure and f / P = 1.

– For a real gas, the fugacity is not equal to P and the ratio f / P varies.

– It is observed, however, that on decreasing the pressure, the behaviour of the gas approaches that of an ideal gas. It may be stated, therefore, that f approaches P as P approaches zero.

– The ratio f / P is called activity coefficient of a gas and is represented by the symbol γ.

– It gives a direct measure of the extent to which any gas deviates from ideal behaviour at any given pressure and temperature for the farther this ratio is from unity, the greater is the non-ideality of the gas.

References