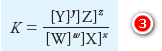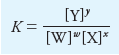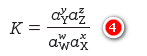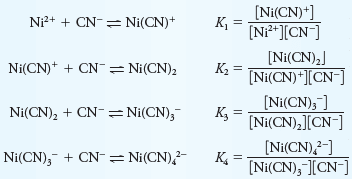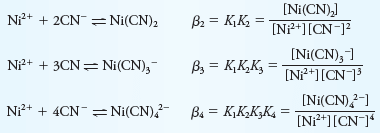Types of Equilibrium Constants used in Analytical Chemistry
– In this subject, we will discuss Types of Equilibrium Constants used in Analytical Chemistry.
Chemical Equilibrium and Equilibrium constants
– Many reactions used in analytical chemistry never result in the complete conversion of reactants to products.
– Instead, they proceed to a state of chemical equilibrium in which the ratio of concentrations of reactants and products is constant.
– Equilibrium constants expressions are algebraic equations that describe the concentration relationships among reactants and products at equilibrium.
– Among other things, equilibrium-constant expressions permit the calculation of the error in an analysis resulting from the quantity of unreacted analyte that remains when equilibrium has been reached.
– In the discussion that follows, we cover the use of equilibrium-constant expressions to gain information about analytical systems in which no more than one or two equilibria are present.
The Equilibrium State
– Consider the chemical reaction:
– We can follow the rate of this reaction and the extent to which it proceeds to the right by monitoring the appearance of the orange-red color of the triiodide ion I3–.
– The other participants in the reaction are colorless
– For example, if 1 mmol of arsenic acid, H3AsO4 is added to 100 mL of a solution containing 3 mmol of potassium iodide, the red color of the triiodide ion appears almost immediately.
– Within a few seconds, the intensity of the color becomes constant, showing that the triiodide concentration has become constant (see color plates 1b and 2b).
– A solution of identical color intensity (and hence identical triiodide concentration) can also be produced by adding 1 mmol of arsenous acid, H3AsO3, to 100 mL of a solution containing 1 mmol of triiodide ion (see color plate 1a).
– Here, the color intensity is initially greater than in the first solution but rapidly decreases as a result of the reaction.
– Ultimately, the color of the two solutions is identical.
– Many other combinations of the four reactants can be used to yield solutions that are indistinguishable from the two just described.
– The results of the experiments shown in color plates 1–3 illustrate that the concentration relationship at chemical equilibrium (that is, the position of equilibrium) is independent of the route to the equilibrium state.
– This relationship is altered by applying stress to the system, however.
– Such stresses include temperature changes, pressure (if one of the reactants or products is a gas), or the total concentration of a reactant or a product.
– These effects can be predicted qualitatively from Le Châtelier’s principle.
Le Châtelier’s Principle
– Le Châtelier’s principle states that the position of chemical equilibrium always shifts in a direction that tends to relieve the effect of applied stress”.
– For example, an increase in the temperature of a system alters the concentration relationship in the direction that tends to absorb heat, and an increase in pressure favors those participants that occupy a smaller total volume.
– In an analysis, the effect of introducing an additional amount of a reactant or product to the reaction mixture is particularly important.
– The resulting stress is relieved by a shift in equilibrium in the direction that tends to use up the added substance.
– Thus, for the equilibrium we have been considering (Equation 1), the addition of arsenic acid (H3AsO4) or hydrogen ions causes an increase in color as more triiodide ion and arsenous acid are formed.
– Adding arsenous acid has the reverse effect.
– An equilibrium shift brought about by changing the amount of one of the participating reactants or products is called a mass-action effect.
– Theoretical and experimental studies of reacting systems on the molecular level show that reactions among the participating species continue even after equilibrium is achieved.
– The concentration ratio of reactants and products is constant because the rates of the forward and reverse reactions are precisely equal.
– In other words, chemical equilibrium is a dynamic state in which the rates of the forward and reverse reactions are identical.
Important Notes on Chemical Equilibrium
(1) The final position of a chemical equilibrium is independent of the route to the equilibrium state.
(2) Le Châtelier’s principle states that the position of an equilibrium always shifts in such a direction as to relieve a stress that is applied to the system.
(3) The mass-action effect is a shift in the position of an equilibrium caused by adding one of the reactants or products to a system.
(4) Equilibrium is a dynamic process. Although chemical reactions appear to stop at equilibrium, in fact, the amounts of reactants and products are constant because the rates of the forward and reverse processes are exactly the same.
(5) Chemical thermodynamics is a branch of chemistry that concerns the flow of heat and energy in chemical reactions. The position of a chemical equilibrium is related to these energy changes.
Equilibrium Constants Expressions
– The influence of concentration or pressure (if the participants are gases) on the position of a chemical equilibrium is conveniently described in quantitative terms by means of an equilibrium-constant expression.
– Equilibrium constant expressions are derived from thermodynamics.
– They are important because they allow us to predict the direction and completeness of chemical reactions.
– An equilibrium-constant expression, however, yields no information concerning the rate of a reaction.
– In fact, we sometimes find reactions that have highly favorable equilibrium constants but are of little analytical use because they are so slow.
– This limitation can often be overcome by the use of a catalyst, which speeds the approach to equilibrium without changing its position.
– Consider a generalized equation for a chemical equilibrium:
– where the capital letters represent the formulas of participating chemical reactants and products, and the lowercase italic letters are the small whole numbers required to balance the equation.
– Thus, the equation says that w moles of W react with x moles of X to form y moles of Y and z moles of Z.
– The equilibrium-constant expression for this reaction is:
– where the square-bracketed terms are:
(1) molar concentrations if they represent dissolved solutes.
(2) partial pressures in atmospheres if they are gas-phase reactants or products.
– In such an instance, we will often replace the square bracketed term (say [Z] in Equation 3) with the symbol pz, which stands for the partial pressure of the gas Z in atmospheres
– If a reactant or product in Equation (3) is a pure liquid, a pure solid, or the solvent present in excess, no term for this species appears in the equilibrium-constant expression.
– For example, if Z in Equation (2) is the solvent H2O, the equilibrium-constant expression simplifies to:
– We discuss the basis for this simplification in the sections that follow.
– The constant K in Equation (3) is a temperature-dependent numerical quantity called the equilibrium constant.
– By convention, the concentrations of the products, as the equation is written, are always placed in the numerator and the concentrations of the reactants are always in the denominator.
– Equation (3) is only an approximate form of a thermodynamic equilibrium constant expression.
– The exact form is given by Equation (4). Generally, we use the approximate form of this equation because it is less tedious and time-consuming.
– where aY, aZ, aW, and aX are the activities of species Y, Z, W, and X
Note:
– Equilibrium constant expressions provide no information about whether a chemical reaction is fast enough to be useful in an analytical procedure
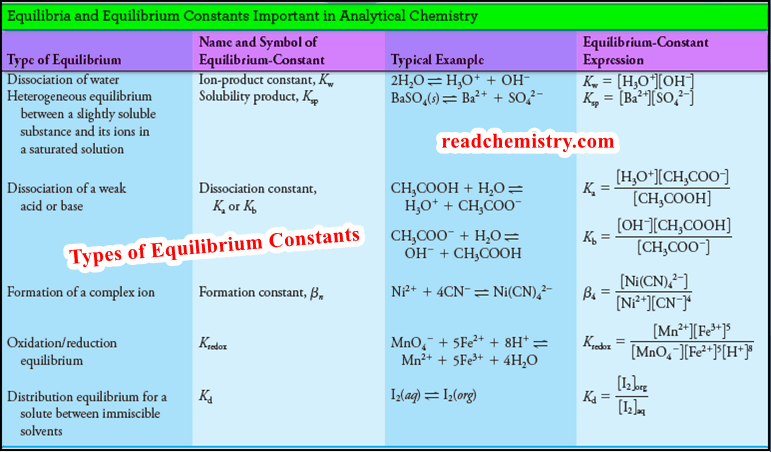
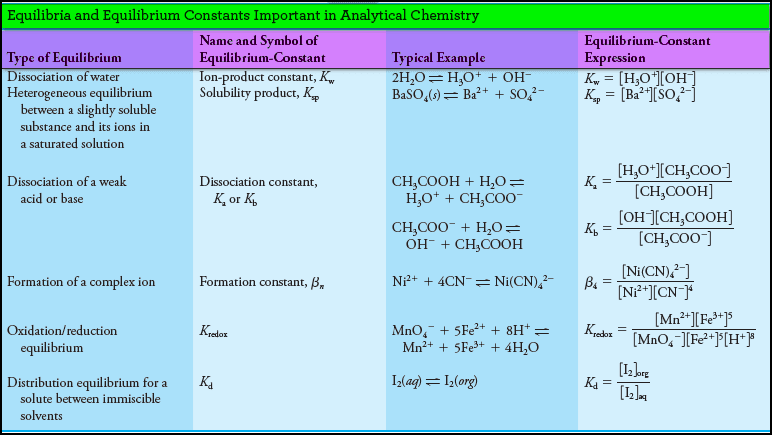
Types of Equilibrium Constants in Analytical Chemistry
– The following Table summarizes the types of chemical equilibria and equilibrium constants that are of importance in analytical chemistry:
Stepwise and Overall Formation Constants for Complex Ions
– The formation of Ni(CN)4-2 (Table 1) is typical in that it occurs in steps as shown.
– Note that stepwise formation constants are symbolized by K1, K2, and so forth
– Overall constants are designated by the symbol βn. Thus,
Reference: Fundamentals of analytical chemistry / Douglas A. Skoog, Donald M. West, F. James Holler, Stanley R. Crouch. (ninth edition) , 2014 . USA